The following question came up in my 245A class today:
Is it possible to express a non-closed interval in the real line, such as [0,1), as a countable union of disjoint closed intervals?
I was not able to answer the question immediately, but by the end of the class some of the students had come up with an answer. It is actually a nice little test of one’s basic knowledge of real analysis, so I am posing it here as well for anyone else who is interested. Below the fold is the answer to the question (whited out; one has to highlight the text in order to read it).
The answer to the question is… no.
First of all, it suffices to prove the claim for an open interval such as (0,1). For, if one partitions a half-open interval such as [0,1) into closed intervals, then after selecting any one of these intervals [a,b], the open interval (b,1) must then also be partitioned into countably many disjoint closed intervals.
By mapping (0,1) homeomorphically to the real line, it suffices to show that the real line R cannot be partitioned into closed intervals.
As each interval is bounded, we need an infinite number of intervals to cover the real line. Now consider the set
consisting of the endpoints of the intervals . Clearly, E is countably infinite. Also, as the
form a disjoint cover of E, E is the complement of the open set
and is hence closed. Finally, we claim that E is perfect: that not only is E closed, but every point in E is a limit point in E. Indeed, if x lies in E, then x is either the left or right endpoint of an interval
, but it is not both. If it is, say, the right-endpoint of an interval, then by approaching x from the right we see that x is the limit of the left endpoint of intervals to the right of x.
Now we appeal to a general theorem (a special case of the even more general Baire category theorem) that asserts that a perfect subset of a complete metric space cannot be countably infinite. A proof is as follows. Suppose for contradiction that is a countably infinite perfect set. Let
be any closed ball of positive radius whose centre lies in E (e.g. one can take the closed ball of radius 1 centered at
). Using the fact that
is a limit point, one can then find a closed ball
inside
whose centre still lies in E, but which is disjoint from
, and whose radius is at most half that of
. (Indeed, if
does not already lie in the interior of
, one simply has to shrink
by a little bit and recenter to another element of E to move
off the boundary of
; otherwise, one uses the limit point property to find another point in the interior of
other than
, and makes a small closed ball around that.) Then one can find a closed ball
inside
of radius at most half that of
whose centre still lies in E, but is now also disjoint from
in addition to
. Continuing in this fashion we find a sequence
of nested closed balls with radii going to zero, each of which has centre in E. The centres of these balls then form a Cauchy sequence, which by completeness, must converge to a limit in E that also lies in
. But by construction,
lies outside each element
of E, and is thus disjoint from E, a contradiction.
Several of the students also came up with a proof based on the fact that the set E had the topological structure of a Cantor set, which is necessarily uncountable. This is close to the proof given above, though the above proof has the advantage that it is more readily generalisable to higher dimensions, showing for instance that a non-closed box cannot be expressed as the countable union of closed boxes. (For the generalisation, one has to use the full strength of the Baire category theorem, rather than just relying on the theory of perfect sets.) UPDATE: as pointed out in comments, the higher-dimensional result can also be easily deduced from the one-dimensional result by looking at a slice.
Note that if one replaces the reals in the question by the rationals, then the answer to the question becomes “yes” (this is easiest to see if one uses closed intervals of zero length, though one can also concoct examples using positive length intervals also). This shows that any proof must use a property of the real line, such as completeness, that is not shared by the rationals, and so it is unlikely that there is any elementary proof that is significantly shorter than the one given above.

59 comments
Comments feed for this article
4 October, 2010 at 2:50 pm
Anonymous
“If it is, say, the __right__-endpoint of an interval, then by approaching x from the right we see that x is the limit of the left endpoint of intervals to the right of x.” [Corrected, thanks – T.]
4 October, 2010 at 3:14 pm
porton
The formulas in this post are completely messed up.
4 October, 2010 at 3:15 pm
porton
Oh, no. It is not messed formulas. It is (in some mysterious reason) invisible text on the page (white on white).
4 October, 2010 at 3:16 pm
porton
Oh, sorry, Terry, for this stupid comment. Now I see that you intentionally whited up the text.
28 September, 2019 at 3:07 am
Anonymous
oh I am still confused about the text
4 October, 2010 at 4:11 pm
Student
Another question that I still dont know how to prove is:
Thm:Show that a union of open intervals can be written as a disjoint union of open intervals.
Induction isn’t enough because induction just works for a finite number of arbitrary open intervals.
I know how where to find the answer, but I want to solve it by myself. I am more than one week trying to solve it. :o)
Best
3 January, 2011 at 6:29 am
Anonymous
I do not have access to Follands book right now, but nevertheless, I tried to proof Students question.
Theorem: Any open subset U of R may be expressed as a disjoint union of open intervals. (this implies his question as any union of open intervals is an open subset of R) [R being the real set]
Proof: Consider the intersection V of U and Q [Q being the rational numbers]. Note that V is countably infinite.
For each q in V, define
A_q = inf{a in U | (a,q) is a subset of U}
B_q = sup{b in U | (q, b) is a subset of U}
Both values exist in R because U is open.
Now, I_q = (A_q, B_q) is an open interval fully contained in U. Because if x is in I_q but not in U, it would contradict our definition of either A_q or B_q.
We obtain a countably infinite amount of intervals I_q, and for q1 and q2 in V we either have
I_q1 = I_q2, or
I_q1 and I_q2 are disjoint. (because if two intervals intersect in any point, they have to be the same by our construction)
Also, because Q is a dense subset of R and U is open, any x in U is an element of I_q for some q in V (consider an open ball around x, and a rational number q within that ball), so we conclude that the union of all I_q equals U.
This may seem sketchy, but being a foreign undergraduate is leaving me with some problems to express myself precisely.
28 September, 2019 at 4:31 am
Anonymous
Any open set in (and in other topological spaces) is a disjoint union of its (open) connected components.
(and in other topological spaces) is a disjoint union of its (open) connected components.
2 June, 2013 at 7:22 am
Gautam
“A Course on Borel Sets” by S M Srivastava. pg.51.
11 August, 2016 at 9:40 am
Anonymous
The intersection of open intervals is open. Deleting the intersection from one of the offending pairs of open intervals gives two open intervals with equal union.
11 August, 2016 at 9:41 am
Anonymous
*one from
11 August, 2016 at 11:04 am
Anonymous
Intersection of open intervals is not necessarily open (e.g. the intersection of all open intervals containing a closed interval).
4 October, 2010 at 9:22 pm
ugroh
Remark to the Student-question: A proof can be found e.g. in Folland, Real Analysis, Prop. 0.21 .
Ulrich
4 October, 2010 at 10:10 pm
David Milovich
Cantor’s original proof of the reals’ uncountability
can be adapted to get a shorter elementary proof.
Start with [0,1) and a sequence (C_n:n=0,1,2,…)
of disjoint closed subintervals of [0,1).
Choose i_0 and j_0 such that C_(i_0)<C_(j_0).
Given C_(i_n)<C_(j_n),
choose the least i_(n+1) such that
C_(i_n)<C_(i_(n+1))<C_(j_n).
Then choose some j_(n+1) such that
C_(i_(n+1))<C_(j_(n+1))<C_(j_n).
Let x be the supremum of the union
of all sets of the form C_(i_n).
Given any n, since C_(i_n)<x<C_(j_n) and i_(n+1) is
minimal with respect to C_(i_n)<C_(i_(n+1))<C_(j_n),
x cannot be in C_k for any k<i_(n+1).
Thus, for all n, x is not in C_k for any k<i_(n+1).
But C_(i_n)<C_(i_(n+1)), so the sequence (i_n:n=0,1,2,…)
never repeats, which implies that the sequence is
unbounded, which implies that x is not in any C_k.
5 October, 2010 at 4:01 am
Marton Eekes
A slightly harder nice exercise is (I only modified the very last word):
Is it possible to express a non-closed interval in the real line, such as [0,1), as a countable union of disjoint closed sets?
5 October, 2010 at 1:52 pm
pavel zorin
Using the hint on the Baire category theorem one could also infer that a complete connected metric space is not a countable union of disjoint closed subsets. I am not sure whether this result generalizes to Baire spaces, though.
best regards, pavel
5 October, 2010 at 3:03 pm
pavel zorin
I have noticed an error in my reasoning: the space should also be locally connected. Nevertheless, I do not have a counterexample if local connectedness does not hold.
7 October, 2010 at 4:51 am
pavel zorin
Here is a counterexample, i.e. a connected complete metric space which is a countable union of closed sets:
Let {e_{i,j}; i>0, j≥0} be orthonormal vectors in a Hilbert space.
Let S_i consist of the closed interval [e_{i,0}, 2 e_{i,0}] and the polylines from 2 e_{i,0} to 2 e_{i,0}+e_{i,j} to e_{j,0}+e_{i,j} to e_{j,0}+e_{i,j}/i.
Then S_i are closed and their union is complete and connected. This set is best visualized as countably many intervals, each of which has antennae in the direction of an endpoint of each other set.
Note that the boundary of each S_i is just {e_{i,0}}, hence the union of the boundaries is a discrete set (as opposed to a perfect set in the case of intervals on the real line)
5 October, 2010 at 10:01 pm
domotorp
I remembered that we used to call such sets $F_\sigma^*$ on the problem solving seminar.
9 October, 2010 at 8:09 pm
Anonymous
I am ignorant of the difference of a closed set and a closed interval of the real line. Can’t any closed set ve decomposed into closed intervals?
5 October, 2010 at 4:59 am
mircea petrache
Take a V-shaped function u:[0,1]->[0,1] such that u(0)=u(1)=1 and u(1/2)=0, and such that u is piecewise affine on [0,1/2], [1/2,1].
Take now a family of intervals which would falsify the theorem. On one such interval [a,b] put v(x)=u((x-a)/(b-a)), then define v(1)=v(0)=1. So from the set of intervals of the text, we got a continuous function v:[0,1]->[0,1], which is =1 just on the union of extrema of the segments.
v is uniformly continuous, but this contradicts the fact that the lenghts of intervals can be arbitrarily small (thus the slope of v is unbounded).
does this make sense?
5 October, 2010 at 9:40 am
Tobias Hagge
Thank you for your wonderful blog. Here is an argument that uses the compactness of closed intervals in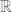 .
.
Given a sequence![([a_i, b_i])_{i\in \mathbb{N}^+}](https://s0.wp.com/latex.php?latex=%28%5Ba_i%2C+b_i%5D%29_%7Bi%5Cin+%5Cmathbb%7BN%7D%5E%2B%7D&bg=ffffff&fg=545454&s=0&c=20201002) of disjoint closed intervals in
of disjoint closed intervals in 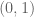 , let
, let ![C_n = \cup_{i=1}^n [a_i,b_i]](https://s0.wp.com/latex.php?latex=C_n+%3D+%5Ccup_%7Bi%3D1%7D%5En+%5Ba_i%2Cb_i%5D&bg=ffffff&fg=545454&s=0&c=20201002) . We’ll construct a nested sequence of nonempty closed intervals
. We’ll construct a nested sequence of nonempty closed intervals 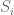 such that
such that 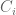 and
and 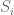 are disjoint. The intersection of such is nonempty and does not intersect any
are disjoint. The intersection of such is nonempty and does not intersect any ![[a_i,b_i]](https://s0.wp.com/latex.php?latex=%5Ba_i%2Cb_i%5D&bg=ffffff&fg=545454&s=0&c=20201002) .
.
Choose![S_0= [c_0,d_0]](https://s0.wp.com/latex.php?latex=S_0%3D+%5Bc_0%2Cd_0%5D&bg=ffffff&fg=545454&s=0&c=20201002) such that
such that  and $a_1 < d_0 < b_1$. If
and $a_1 < d_0 < b_1$. If ![[a_i,b_i]](https://s0.wp.com/latex.php?latex=%5Ba_i%2Cb_i%5D&bg=ffffff&fg=545454&s=0&c=20201002) is disjoint from
is disjoint from  , let
, let 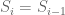 . Otherwise, suppose that
. Otherwise, suppose that 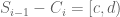 . This lies in some component
. This lies in some component 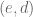 of the complement of
of the complement of 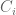 in
in 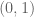 . Set
. Set ![S_i = [c_i,d_i]](https://s0.wp.com/latex.php?latex=S_i+%3D+%5Bc_i%2Cd_i%5D&bg=ffffff&fg=545454&s=0&c=20201002) , where
, where  , chosen so that the least-indexed $[a_i,b_i]$ which lies in
, chosen so that the least-indexed $[a_i,b_i]$ which lies in 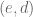 contains
contains 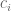 but not
but not  . The case
. The case ![S_{i-1} - C_i = (c,d]](https://s0.wp.com/latex.php?latex=S_%7Bi-1%7D+-+C_i+%3D+%28c%2Cd%5D&bg=ffffff&fg=545454&s=0&c=20201002) is similar.
is similar.
5 October, 2010 at 1:43 pm
Danny Calegari
I think this is a theorem of Sierpinski; the reference I have in mind is “Un theoreme sur les continus” (Tohoku Math 13 (1918) 300-303) but I don’t have the reference handy.
5 October, 2010 at 4:22 pm
Top Posts — WordPress.com
[…] Covering a non-closed interval by disjoint closed intervals The following question came up in my 245A class today: Is it possible to express a non-closed interval in the real […] […]
5 October, 2010 at 9:25 pm
Tobias Hagge
One may give a purely topological proof by constructing a nested sequence of closed intervals S_n, the intersection of which is disjoint from the union of the I_n. Suppose S_n is chosen so that for the least k such that I_k is not disjoint from S_n, S_n – I_k is a half-open interval. Inside of this half-open interval is a closed subinterval S_{n+1} with the same property and larger least k.
This gives the result for any dense total order on which closed intervals are compact. However, I’m having a little trouble comparing the generality of that argument to the argument in the post. Are such spaces always metrizable?
7 October, 2010 at 1:33 pm
Tobias Hagge
Why the down vote?
11 October, 2010 at 10:51 am
Tobias Hagge
To answer my own question, no. Counterexample: the hyperreals. More general counterexample: the Dedekind-MacNeille completion of any non-metrizable total order. By the least upper bound property, closed intervals are compact. The completion is not metrizable since it has a non-metrizable subspace.
6 October, 2010 at 6:54 am
gowers
In Cambridge this is a well-known “exercise for the enthusiast” that has been tackled by several generations of the brighter undergraduates.
As a little experiment, I just typed “Can [0,1] be covered by countably many disjoint closed intervals?” into Google (with no inverted commas) and this post came up first. So now there will be an easy way of solving the problem. I wonder if eventually this method will work for all exercises. (I’ve just tried it for one or two other questions and it didn’t work, so we haven’t reached this state yet.)
6 October, 2010 at 5:32 pm
anonymous
No, in fact we have the opposite problem: it’s often inconveniently difficult to find the answers to basic mathematical questions by searching online.
(I had a rare success with this the other day when looking for an example of a differentiable function that isn’t continuously differentiable. After a surprisingly long few minutes I found what I was looking for, but in general this is the type of thing that is difficult to find: things that “everyone knows” but that don’t have standard names.)
6 October, 2010 at 7:08 am
Xueping
I have a different proof which I can’t judge whether it’s available.
Observation: Let $ (a_n, b_n)$ be a strictly decreasing sequence of open intervals. If $a_n < a_{n+1}< b_{n+1}<b_{n}$ is always true, then their intersection is not empty.
Suppose that $(0,1)= \cup [c_n , d_n]$, where $ [c_n , d_n]$ are disjoint. Take $A_0 =(0,1)$.
Consider the complement of $[c_1 ,d_1]$, $(0, c_1) \cup (c_2, 1)$, $[c_2, d_2]$ must lie in one of the two intervals, say $(0, c_1) $ for example.
Then take $A_1 =(d_2 , c_1)$. Wait until the next $[c_i, d_i]$ lies in $A_1$ and another $[c_k, d_k]$ divides an interval of the complement of $[c_i, d_i]$ in $A_1$. Then in the resulting two intervals, choose one that has no common endpoints with $A_1$ to be $A_2$. Inductively we can get a strictly decreasing sequence of open intervals $A_n$, which satisfies that the endpoints never coincide. As a consequence, the intersection of them is nonempty.
From the construction, we can see that this intersection is a subset of the complement $\cup [c_n , d_n]$, which is empty. A contradiction.
6 October, 2010 at 7:19 am
huangxueping
I have a different proof which I can’t judge whether it’s available. be a strictly decreasing sequence of open intervals. If
be a strictly decreasing sequence of open intervals. If 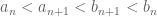 is always true, then their intersection is not empty.
is always true, then their intersection is not empty.
Observation: Let
Suppose that![(0,1)= \cup [c_n , d_n]](https://s0.wp.com/latex.php?latex=%280%2C1%29%3D+%5Ccup+%5Bc_n+%2C+d_n%5D&bg=ffffff&fg=545454&s=0&c=20201002) , where
, where ![[c_n , d_n]](https://s0.wp.com/latex.php?latex=%5Bc_n+%2C+d_n%5D&bg=ffffff&fg=545454&s=0&c=20201002) are disjoint. Take
are disjoint. Take  .
.![[c_1 ,d_1]](https://s0.wp.com/latex.php?latex=%5Bc_1+%2Cd_1%5D&bg=ffffff&fg=545454&s=0&c=20201002) ,
, 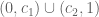 ,
, ![[c_2, d_2]](https://s0.wp.com/latex.php?latex=%5Bc_2%2C+d_2%5D&bg=ffffff&fg=545454&s=0&c=20201002) must lie in one of the two intervals, say
must lie in one of the two intervals, say 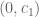 for example.
for example. . Wait until the next
. Wait until the next ![[c_i, d_i]](https://s0.wp.com/latex.php?latex=%5Bc_i%2C+d_i%5D&bg=ffffff&fg=545454&s=0&c=20201002) lies in
lies in 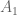 and another
and another ![[c_k, d_k]](https://s0.wp.com/latex.php?latex=%5Bc_k%2C+d_k%5D&bg=ffffff&fg=545454&s=0&c=20201002) divides one interval of the complement of
divides one interval of the complement of ![[c_i, d_i]](https://s0.wp.com/latex.php?latex=%5Bc_i%2C+d_i%5D&bg=ffffff&fg=545454&s=0&c=20201002) in
in 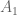 into two intervals . Then in the resulting two intervals, choose one that has no common endpoints with
into two intervals . Then in the resulting two intervals, choose one that has no common endpoints with 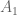 to be
to be 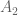 . Inductively we can get a strictly decreasing sequence of open intervals
. Inductively we can get a strictly decreasing sequence of open intervals 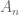 , which satisfies that their endpoints never coincide. As a consequence, the intersection of them is nonempty.
, which satisfies that their endpoints never coincide. As a consequence, the intersection of them is nonempty.![\cup [c_n , d_n]](https://s0.wp.com/latex.php?latex=%5Ccup+%5Bc_n+%2C+d_n%5D&bg=ffffff&fg=545454&s=0&c=20201002) , which is empty. A contradiction.
, which is empty. A contradiction.
Consider the complement of
Then take
From the construction, we can see that this intersection is a subset of the complement
9 October, 2010 at 2:12 am
Leena
:huangxueping I am sorry but I feel this does not complete the proof… cuz a closed set in (0,1) need not necessarily be in the form [c,d] it could be something like {a,b,c,d} etc…The above proof proves that (0,1) can not be written as a union of disjoint connected and closed subsets of (0,1). I guess We need a more general prof for the above thm.
29 July, 2013 at 1:45 pm
Fan
I think this proof also generalizes to the case of closed sets. Consider the complement of each closed set, which is open and can be written as a countable union of open intervals. It is easy to see that there cannot be only one open interval (since the complement is not connected), so you still have the freedom of choice to make a nested sequence of open intervals whose intersection is not empty.
29 July, 2013 at 1:51 pm
Fan
Is this proof morally the same as Hagge’s?
6 October, 2010 at 5:01 pm
J Balachandran
I might be misunderstanding the question. If I’m correct a finite union of closed sets in a closed set. However the given set is not closed since the limit point 1 does not belong to the set. So there is no way we can have a closed set to represent this non-closed set. Is this reasoning correct? If wrong can someone highlight me where the mistake is
6 October, 2010 at 9:52 pm
Anonymous
Not quite. The question is whether the open set can be expressed as a *countable* union of closed sets (ie infinite unions are allowed). Your explanation only holds if we are constrained to a *finite* union of closed sets.
7 October, 2010 at 4:52 am
J Balachandran
Oh ok. sorry. I got the mistake
6 October, 2010 at 8:38 pm
Jonathan Weinstein
J Balachandran: It’s a countable union, not finite, so not necessarily closed.
Huang Xueping: Very nice. You ruined my day though, I solved it your way and scrolled through the first 15 comments without seeing this…oh well.
9 October, 2010 at 2:05 am
Leena
but the sets are disjoint… so even if it is a countable union and not finite … it is still a closed set….. if we take its closure … we realize its a closed set.
12 October, 2010 at 6:36 pm
Will
What about intervals of the form
[1/(2^(2n+1)),1/(2^(2n)]
Take the union (for natural numbers n) of all such intervals and 0 is a limit point. Thus, a countable union of disjoint closed intervals need not be a closed set.
6 October, 2010 at 8:44 pm
Apoorva Khare
First, Huang Xueping’s proof can basically be modified to work for the more general question of covering (or not) by closed SETS (posed by Marton Eekes).
Second, to generalize this to higher dimensions (can the open unit ball be covered by countably many disjoint closed SETS in Euclidean space?), is it not enough to intersect with the “X-axis”? For if there were such a cover, then the intersection with the X-axis would give a countable cover of (-1,1) by disjoint closed sets, which is false.
[Good point; I’ve added a mention of this in the post. -T.]
6 October, 2010 at 8:49 pm
Apoorva Khare
Curiously, I first saw the (more general, ref. Eekes) problem eight years ago in graduate school (and I solved it essentially by Huang Xueping’s method too), as well as the “higher dimensions” variant. Just recently, I asked it of my students here at Yale, some three weeks ago! Then I posed it to a friend in Ann Arbor, and he asked it of his students 1-2 weeks ago. Curiously, the same problem showed up in Prof. Tao’s class in UCLA this week.
7 October, 2010 at 12:29 am
Koen van Woerden
Suppose a countable cover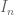 of closed disjoint sets of the interval
of closed disjoint sets of the interval 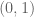 exists.
exists.
Define the function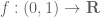 as follows: each
as follows: each  in
in 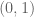 is covered by precisely one
is covered by precisely one 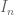 . Send
. Send  to
to  . Then
. Then  is continuous, and since the image of $f$ is contained in the natural numbers, by the intermediate value theorem
is continuous, and since the image of $f$ is contained in the natural numbers, by the intermediate value theorem  is constant. Hence
is constant. Hence 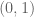 is covered by a single closed interval. Hence
is covered by a single closed interval. Hence  is closed. Contradiction.
is closed. Contradiction.
7 October, 2010 at 12:34 am
Koen van Woerden
Never mind, this is not a good proof. Sorry for that.
7 October, 2010 at 4:15 am
Liu Shiping
Also, as the $I_n$ form a disjoint partition of E,
I guess it should be a disjoint partition of the real line.
[Corrected, thanks – T.]
7 October, 2010 at 2:27 pm
some graduate student
This confused me too. Usually a “partition” is a collection of subsets of the set satisfying certain conditions — but the I_n aren’t subsets of E.
8 October, 2010 at 12:13 am
mircea petrache
I try to make a proof like Koen van Woerden’s, i.e. using functions and continuity. It looks elementary just because the key points are left to the reader.
Take a square [0,1]x[-1,0], and consider the inscribed semicircle with diameter the “upper” side [0,1]x{0}. Now suppose a family as in the theorem exists. Then for each segment I in the family, put a shrinked copy of the above square. Then the union of the “shrinked semicircles” will describe the graph of a continuous function (since at the end points of any segment, shorter segments accumulate from one side).
But this also would define a continuous function on [0,1] (if you extend to zero at the extrema). Thus it should also be uniformly continuous. Still, the fact that the length of segments is arbitrarily close to 0 (and the fact that the tangent to the semicircles is vertical), contradicts any choice of modulus of continuity.
8 October, 2010 at 12:17 am
mircea petrache
again the proof was wrong, maybe stretching the vertical direction of the squares to square root of I makes it work
8 October, 2010 at 12:53 am
Vladimir Slepnev
I got the answer “no” by the following argument that sounds very natural to me: 1) reduce the problem to the open interval like you did, 2) removing a closed interval turns an open interval into two open intervals, 3) repeating the process countably many times leaves us with a binary tree of intervals, 4) if some interval never gets split then we have our answer, 5) if all intervals get split then alternating left-right-left-right-… gives us an intersection point. Completeness is used in the last step.
8 October, 2010 at 6:04 pm
Arindam Bandyopadhyay
Dear Sir,
Please discuss about the following problem:
Show that $\{\sum\limits_{k=0}^{n}\frac{1}{k^{2}}\}$ is a Cauchy sequence by using the following definition of Cauchy sequence:
A sequence $\{x_{n}\}$ is said to be a Cauchy sequence if for any $\epsilon >0$, there exists a $m\in\mathbb{N}$ such that $|x_{n+p}-x_{n}|<\epsilon$ for all $n\geq m$ and all $p\in\mathbb{N}$.
8 October, 2010 at 9:44 pm
Leena
let (0,1) = union of countable union of I(n) where each I (n) is closed and all of them are disjoint. Then denote (0,1) by set A and the countable union of I(n) as B. We claim sets A and B are equal. then Closures of both of them also must be equal.. closure of (0,1) is [0,1]. Now taking the closure of the countable union of I(n) …. all the sets are disjoint and hence closure of union of I (n) is equal to union of closures of each of I (n). but all of I (n) are closed. So its the same set B again. Hence the closure of A is not equal to closure of B . Which is a contradiction. so (0,1) can not be written as a union of countable union of disjoint closed sets. Its applicable to all open intervals too…
10 October, 2010 at 8:24 am
Lewis Bowen
I think I found a different proof. It begins the same way; it suffices to show that the real line cannot be covered by countably many disjoint intervals. Suppose for a contradiction that it can. Let C_1, C_2,… be such a covering. Let O_i be the union of the complement of C_i with the interior of C_i (so O_i is the whole line minus the two endpoints of C_i). So O_i is a dense open set. Accordingly the intersection of all the O_i’s is a dense G_delta. But it must be empty if the C_i’s cover and are disjoint. This contradiction proves it.
11 November, 2010 at 9:33 am
Local Connectedness and Some Weird Counterexamples « Gracious Living and the Two Meat Meal
[…] only way to do this is by letting itself be the only one in the union; proofs are, for example, here (read the comments for other viewpoints/try it yourself first!). So the only paths in the set are […]
17 March, 2011 at 1:51 pm
Manuel
To prove that [0,1) can’t be expressed as a union of countable many disjoint closed intervals, can’t we just say de following?
Suppose we can. We have then a countable family F of closed intervals, which can be ordered in the sense reals are (?). We order them creating the sequence {[a_n, b_n]}, where b_1<a_2, b_2<a [text lost here due to unintended HTML processing of the < and > signs – T.] >b_k (since disjoint). So we can find some c in (b_k, a_(k+1)) opened set which hasn’t been covered by the family F.
Maybe there’s an error when saying we can order that family F, because if not this would sound good to me…
22 September, 2011 at 7:00 am
Sergei Ovchinnikov
See American Mathematical Monthly, 84 (1977),827-828.
29 July, 2013 at 5:07 am
Luqing Ye
I feel frustrated to see that the answer is “no” because this means that my intuition is not reliable when faced with infinity…
Which means I need to adjust my intuition.
Now I give a proof to show why it is impossible to cover a non-closed interval by countable disjoint closed intervals.
In order to prove that it is impossible to cover [0,1) by countable disjoint closed intervals,it is clear that we just need to prove that it is impossible to cover [0,1] by countable disjoint closed intervals.
We prove by contradiction.
Otherwise,
There must exists a sequence of real numbers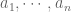 in [0,1] which is convergent to a real number in [0,1],and every item in this sequence is exactly covered by one non-degenerate closed interval(we call the interval of the shape [a,a] as the degenerate closed interval),and any of the two non-degenerate closed intervals are disjoint.
in [0,1] which is convergent to a real number in [0,1],and every item in this sequence is exactly covered by one non-degenerate closed interval(we call the interval of the shape [a,a] as the degenerate closed interval),and any of the two non-degenerate closed intervals are disjoint.
This is impossible,because at the very end of this sequence,two items will be very near that they must be in one non-degenerate intervals.
15 August, 2013 at 12:49 am
Luqing Ye
Dear Prof.Tao,
I don’t know whether you regard [1,1] as a closed interval or not.
9 November, 2021 at 2:29 am
Anonymous
Since it is singleton it is close
29 April, 2015 at 8:11 am
myc10003
I have a different proof. Consider an arbitrary covering of (0, 1) by pairwise disjoint, closed intervals I_x, each I_x is in (0, 1). Denote the covering set by L. One can show that L is a linear continuum and hence uncountable.
Since the closed intervals are pairwise disjoint, there is a natural total order amongst them. Since L covers (0, 1), for any I_x < I_y in L, there exists an I_z in L such that I_x < I_z < I_y.
It remains to show that L has the least upper bound property. Suppose a subset of L, S, is bounded above by an I_x. There must exist a least upper bound of the right endpoints of the intervals in this subset. Call it a. Obviously a is either to the left of I_x or in I_x. Hence a is in (0, 1). If a is the right endpoint of an interval in S, that interval is the least upper bound of S. Otherwise, there exists an I_y in L – S that covers a. Since a is the least upper bound of the right ends of intervals in S, a must be the left endpoint of I_y. Hence I_y is the least upper bound. Therefore L has the least upper bound property.