Last week, we had Peter Scholze give an interesting distinguished lecture series here at UCLA on “Prismatic Cohomology”, which is a new type of cohomology theory worked out by Scholze and Bhargav Bhatt. (Video of the talks will be available shortly; for now we have some notes taken by two note–takers in the audience on that web page.) My understanding of this (speaking as someone that is rather far removed from this area) is that it is progress towards the “motivic” dream of being able to define cohomology for varieties
(or similar objects) defined over arbitrary commutative rings
, and with coefficients in another arbitrary commutative ring
. Currently, we have various flavours of cohomology that only work for certain types of domain rings
and coefficient rings
:
- Singular cohomology, which roughly speaking works when the domain ring
is a characteristic zero field such as
or
, but can allow for arbitrary coefficients
;
- de Rham cohomology, which roughly speaking works as long as the coefficient ring
is the same as the domain ring
(or a homomorphic image thereof), as one can only talk about
-valued differential forms if the underlying space is also defined over
;
-adic cohomology, which is a remarkably powerful application of étale cohomology, but only works well when the coefficient ring
is localised around a prime
that is different from the characteristic
of the domain ring
; and
- Crystalline cohomology, in which the domain ring is a field
of some finite characteristic
, but the coefficient ring
can be a slight deformation of
, such as the ring of Witt vectors of
.
There are various relationships between the cohomology theories, for instance de Rham cohomology coincides with singular cohomology for smooth varieties in the limiting case . The following picture Scholze drew in his first lecture captures these sorts of relationships nicely:
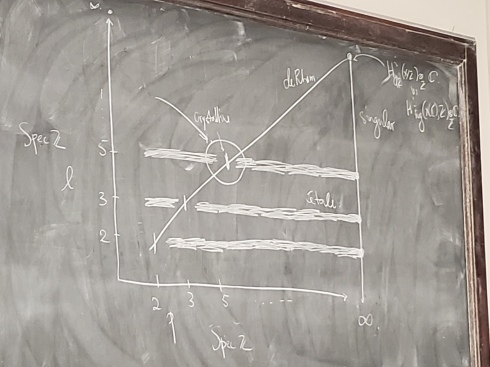
The new prismatic cohomology of Bhatt and Scholze unifies many of these cohomologies in the “neighbourhood” of the point in the above diagram, in which the domain ring
and the coefficient ring
are both thought of as being “close to characteristic
” in some sense, so that the dilates
of these rings is either zero, or “small”. For instance, the
-adic ring
is technically of characteristic
, but
is a “small” ideal of
(it consists of those elements of
of
-adic valuation at most
), so one can think of
as being “close to characteristic
” in some sense. Scholze drew a “zoomed in” version of the previous diagram to informally describe the types of rings
for which prismatic cohomology is effective:

To define prismatic cohomology rings one needs a “prism”: a ring homomorphism from
to
equipped with a “Frobenius-like” endomorphism
on
obeying some axioms. By tuning these homomorphisms one can recover existing cohomology theories like crystalline or de Rham cohomology as special cases of prismatic cohomology. These specialisations are analogous to how a prism splits white light into various individual colours, giving rise to the terminology “prismatic”, and depicted by this further diagram of Scholze:

(And yes, Peter confirmed that he and Bhargav were inspired by the Dark Side of the Moon album cover in selecting the terminology.)
There was an abstract definition of prismatic cohomology (as being the essentially unique cohomology arising from prisms that obeyed certain natural axioms), but there was also a more concrete way to view them in terms of coordinates, as a “-deformation” of de Rham cohomology. Whereas in de Rham cohomology one worked with derivative operators
that for instance applied to monomials
by the usual formula
prismatic cohomology in coordinates can be computed using a “-derivative” operator
that for instance applies to monomials
by the formula
where
is the “-analogue” of
(a polynomial in
that equals
in the limit
). (The
-analogues become more complicated for more general forms than these.) In this more concrete setting, the fact that prismatic cohomology is independent of the choice of coordinates apparently becomes quite a non-trivial theorem.

22 comments
Comments feed for this article
19 March, 2019 at 8:55 am
Daniel Grieser
Thanks, nice post, just one thing: the name is Scholze, not Schölze (no Umlaut here).
Best, Daniel
>
[Corrected, thanks. No idea why I thought there was an umlaut, especially given that I know how to pronounce his name… -T.]
19 March, 2019 at 10:25 am
anon
Still a couple of extra umlauts, just above the second and third images.
[Corrected, thanks – T.]
19 March, 2019 at 11:08 am
Anonymous
Nice post! Two things that didn’t read right:
“and with coefficients in another arbitrary commutative rings”
“which a remarkably powerful application of étale cohomology”
[Corrected, thanks – T.]
19 March, 2019 at 6:20 pm
Will Sawin
Some minor quibbles:
The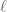 -adic cohomology has (unsurprisingly) coefficients in the
-adic cohomology has (unsurprisingly) coefficients in the 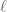 -adic numbers
-adic numbers  , which are not really of characteristic
, which are not really of characteristic 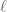 . Instead, as you note later, it is a slight deformation of
. Instead, as you note later, it is a slight deformation of 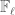 , and it is “close to characteristic
, and it is “close to characteristic 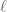 “. This seems worth mentioning – I would say “works well when
“. This seems worth mentioning – I would say “works well when  is the
is the 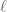 -adic numbers for
-adic numbers for 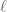 different form the characteristic of
different form the characteristic of  “.
“.
All these cohomology theories, if they work over a ring , also work over any ring
, also work over any ring  that admits a map from
that admits a map from  , because we can tensor over
, because we can tensor over  with
with  . So de Rham cohomology works over any ring which admits a map from
. So de Rham cohomology works over any ring which admits a map from  , etc. I am not sure whether this is worth mentioning.
, etc. I am not sure whether this is worth mentioning.
The formula for the -derivative of
-derivative of  should have
should have  , not
, not  .
.
And this one is just for fun:
Peter Woit pointed out in Bhargav Bhatt’s Eilenberg’s lectures on prismatic cohomology that this diagram of a prism does not accurately reflect the behavior of light in a prism (Some of the light is deflected in the wrong direction as it enters the prism).
[Corrected, thanks – T.]
20 March, 2019 at 9:33 am
Peter Woit
Will,
I don’t remember that, I strongly suspect you’re giving me undeserved credit for someone else’s astute observation.
21 March, 2019 at 1:11 am
Will Sawin
You’re probably right as I am pretty bad at names, faces, and generally distinguishing one human being from another. I apologize for giving you more credit than you deserve.
20 March, 2019 at 6:09 pm
Marni Dee Sheppeard
I would really be very interested to know where they got these ideas from. I have been working on ‘motivic quantum gravity’ for many years, as a category theorist physicist. Last year I spent some months in Los Angeles working with Michael Rios and other people associated to UCLA. (But as a target of abuse, I am now homeless again.) Michael and I were/are both very well aware that the axioms for cohomology underpin quantum gravity.
21 March, 2019 at 9:57 am
Terence Tao
As far as I can tell, the original paper is that of Bhatt, Scholze, and Morrow in https://arxiv.org/abs/1602.03148 . At that stage they did not have a good name for their cohomology theory and called it something like a “q-deformation of de Rham cohomology”. But they conjectured that there was a site-theoretic foundation of this cohomology theory (see Remark 1.11 and footnote 10 of that paper), and when Bhatt and Scholze found that site, it resembled the shape of a prism geometrically, leading to their dubbing of it the “prismatic site”, and the renaming of their theory as “prismatic cohomology”. As mentioned in the post, this nomenclature was also influenced by the famous Pink Floyd album cover.
21 March, 2019 at 6:33 pm
Marni Dee Sheppeard
Thank you. What I am actually wondering about is the unit axioms in higher dimensional categories (notably tricategories a la Gordon, Power, Street). If we can make sense of the associahedra (in the physical axioms) then the next task is to understand the prisms and other topes that show up.
23 March, 2019 at 9:57 am
Anonymous
Marni: There is no actual mathematical connection between Bhatt-Scholze’s work and whatever “motivic quantum gravity” is. You’re latching onto accidental similarities between terminology and using them to insinuate something nefarious. In my experience, Peter Scholze has always been remarkably generous with his ideas and is always careful to give ample credit to other people.
23 March, 2019 at 12:26 pm
Marni Dee Sheppeard
Anonymous coward, no. I am not stupidly latching onto anything. Motivic quantum gravity has q deformations at its heart, starting with fusion categories for quantum computation.
23 March, 2019 at 2:15 pm
Anonymous (but not a coward)
Marni: Scholze’s work has nothing to do quantum gravity or quantum computation or fusion categories (or other such related things), but rather with subtle arithmetic and topological properties of schemes. And I didn’t use the word “stupid” when describing you. Please don’t put words in my mouth.
23 March, 2019 at 4:07 pm
Marni Dee Sheppeard
I never said Scholze had a history of working on quantum gravity, because he clearly does not. However, many people who work on motivic math phys (eg. Brown, me) are quite convinced that it has a lot to do with quantum field theory, and probably also quantum gravity. My comments might well draw Scholze’s attention to a wide literature with which he was not previously familiar, in which case they are worthwhile. (And insulting people without proving any expertise of your own is a cowardly act – every time).
20 March, 2019 at 6:14 pm
Marni Dee Sheppeard
Moreover, Michael is well known at UCLA for wearing Pink Floyd T-shirts while talking about (our) theory of quantum gravity. He also used the prism on arxiv papers last year.
25 March, 2019 at 7:15 pm
Some Quick Items | Not Even Wrong
[…] Peter Scholze’s lecture series at UCLA on Prismatic Cohomology, discussed by Terry Tao here. In related news, this week at MSRI there’s an interesting workshop on Derived Algebraic […]
28 March, 2019 at 11:52 am
Maths student
Hang on, “dark side of the moon”?
2 April, 2019 at 11:16 am
Maths student
Also, given that the moon is a sphere, it seems to be a bit of a stretch to talk about a “side” in this context.
2 April, 2019 at 11:47 am
Anonymous
The word “moon” appears also in the mathematical “moonshine theory” connecting between groups, modular forms and string theory.
6 April, 2019 at 11:48 am
Ricardo Menares Valencia
Tank you very much for this post!
A minor comment: pZ_p is not a subring of Z_p, because pZ_p is not a ring (it has no unit). Rather, it is a maximal ideal such that the quotient Z_p/pZ_p is a field of characteristic p. In this kind of situations, people usually talk about “mixed characteristic” (the characteristic of the residue field is different form the characteristic of the ring).
[Corrected, thanks -T.]
24 July, 2019 at 10:57 pm
Martin Lotz (@mlotzo)
A minor technical comment: you should clean your blackboards :-) Nice summary though.
1 May, 2020 at 9:38 am
Rex
Is there any reason why Scholze’s lectures are not downloadable from the UCLA DLS website? It is hosted on box.com and there is no button to download. But there is one for all other DLS lecturers on box.com.
19 January, 2021 at 10:15 am
课程视频区 – Langlands Program
[…] A great preview of those lectures was given by Tao’s blog https://terrytao.wordpress.com/2019/03/19/prismatic-cohomology/. 一个很不错的笔记见 […]